XiaoMi-AI文件搜索系统
World File Search System汉堡的“ Manfred Eigen校园”
来源:1 Haakenstad,A。,Et。al。(2022)。在1990 - 2019年评估204个国家和领土的总体和特定年龄段的医疗保健访问和质量指数的表现:2019年全球疾病负担研究的系统分析。柳叶刀全球健康,[在线] 10(12),pp.E1715 – E1743。doi:https://doi.org/10.1016/s2214-109x(22)00429-6。2 Bergen,P。等。 al。 (2023)。 平衡制药投资,访问和更健康的生活。 [在线] ZS Insights。 可在以下网址提供:https://www.zs.com/insights/zs-research-global-disease-barancing-balancing-pharma investment。2 Bergen,P。等。al。(2023)。平衡制药投资,访问和更健康的生活。[在线] ZS Insights。可在以下网址提供:https://www.zs.com/insights/zs-research-global-disease-barancing-balancing-pharma investment。
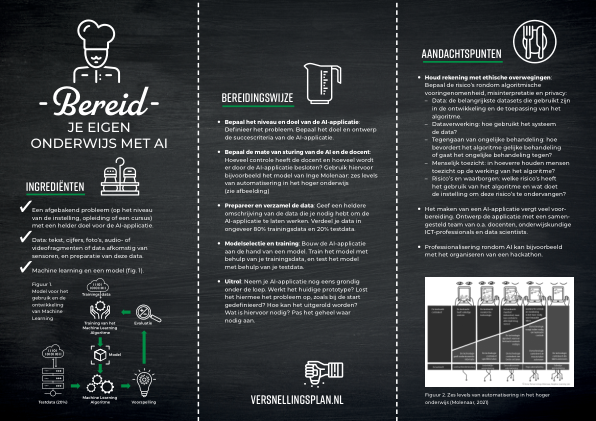
利用人工智能进行自我教育
“人工智能”(AI)一词由科学家约翰·麦卡锡于 1956 年提出。他将人工智能定义为“制造智能机器的科学和工程”。目前存在的系统可以在大量数据中发现模式,并复制人类的思维和理性推理,例如概括、争论、解释和从过去学习。人们可以利用这些系统来执行各种任务,这些系统甚至可以接管某些任务。
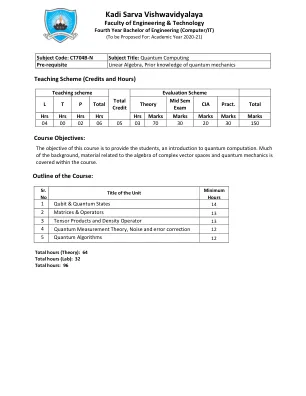
模块2:量子力学(CSE流)
波粒二元论DeBroglie假设(衍生和不同形式的波长)物质波及其特性(相位速度波数据包,群体速度和物质波的群体和特性)HeisenbergHeisenberg的不确定性原理(陈述和说明)和不确定性的prince crordiationprinc prind criventerprinc print crive of prinction Function and Time Independent Schrödinger Wave Equation (Meaning of wave function and differential wave equation for matter in 1-dimention Physical significance of Wave Function: Physical Interpretation (Probability density and normalization) Expectation Value in quantum mechanics (Definition and example) Eigen values and eigen functions (Meaning and conditions for Eigen functions) Applications of schrödinger wave equation: Particle in one-dimensional potential well of infinite height (Applying Schrodinger wave equation and boundary conditions for particle and discussion of Eigen values and Eigen functions) Wave functions and the probability densities for the first three values of for a particle in a box (Using Eigen function, for n=1, 2, and 3, probability density and discussion about the wave nodes) Numerical Problems: Problems on de-Broglie hypothesis, uncertainty principle, expectation value, Eigen value and特征功能预期模型问题:预期问题和上学期结束考试问题。
M.Tech。机械设计 2020-22 - 从来没有
模块 II:线性代数 - 2 特征值和特征向量,特征值的界限 - 盖尔施戈林圆定理。吉文方法、对称矩阵对角化的雅可比方法、任意矩阵的鲁蒂豪瑟方法、幂方法、逆幂方法(SLE:获取特征值和特征向量的分析方法)。
CT704B-N量子计算.pdf
Matrices & Operators Observables, The Pauli Operators, Outer Products, The Closure Relation, Representation of operators using matrices, outer products & matrix representation, matrix representation of operators in two dimensional spaces, Pauli Matrix, Hermitian unitary and normal operator, Eigen values & Eigen Vectors, Spectral Decomposition, Trace of an operator, important properties of Trace, Expectation Value of Operator, Projection操作员,正算子,换向器代数,海森堡不确定性原理,极性分解和奇异值,量子力学的假设。
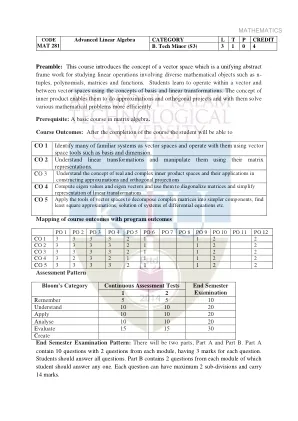
序言:本课程介绍了向量空间的概念,该矢量空间是一项统一的抽象框架,用于研究涉及潜水员的线性操作
co 1将许多熟悉的系统视为向量空间,并使用矢量空间工具(例如基础和维度)与它们一起运行。co 2了解线性变换并使用其矩阵表示来操纵它们。CO 3 Understand the concept of real and complex inner product spaces and their applications in constructing approximations and orthogonal projections CO 4 Compute eigen values and eigen vectors and use them to diagonalize matrices and simplify representation of linear transformations CO 5 Apply the tools of vector spaces to decompose complex matrices into simpler components, find least square approximations, solution of systems of differential equations etc.
5-syllabus-1700469682.pdf
单位 - I:通过梯形形式和正常形式的矩阵矩阵等级,高斯 - 约旦方法的非单个矩阵倒数,线性方程系统:求解高斯消除方法的均匀和非均匀方程的系统,高斯·塞德尔迭代方法。UNIT - II: Eigen values and Eigen vectors Linear Transformation and Orthogonal Transformation: Eigen values, Eigenvectors and their properties, Diagonalization of a matrix, Cayley-Hamilton Theorem (without proof), finding inverse and power of a matrix by Cayley -Hamilton Theorem, Quadratic forms and Nature of the Quadratic Forms, Reduction of Quadratic form通过正交转换为规范形式。单元-III:微积分平均值定理:Rolle的定理,Lagrange的平均值定理,其几何解释和应用,Cauchy的平均值定理,Taylor的系列。确定积分的应用在评估曲线旋转的表面区域和体积(仅在笛卡尔坐标中),不当积分的定义:beta和伽马功能及其应用。单位-IV:多变量计算(部分分化和应用)的定义极限和连续性。部分区分:Euler的定理,总导数,Jacobian,功能依赖性和独立性。应用程序:
提出的教学大纲 - 学期
Matrix Algebra: Types of Matrices, Inverse of a matrix by elementary transformations, Rank of a matrix (Echelon & Normal form), Linear dependence, Consistency of linear system of equations and their solution, Characteristic equation, Eigen values and Eigen vectors, Cayley-Hamilton Theorem, Diagonalization, Complex and Unitary Matrices and its properties.4个多个积分:双重和三个积分,集成顺序的变化,变量的变化,集成在长度,表面积和体积上的应用 - 笛卡尔和极性坐标。beta和伽马功能,Dirichlet的积分及其应用。5向量计算:矢量的点功能,梯度,差异和卷曲及其物理解释,矢量身份,切线和正常定向衍生物。线,表面和音量积分,Green's,Stoke's和Gauss Divergence定理的应用。
基础工程科学系
等级,正常形式,线性方程系统,线性独立和依赖的向量,矩阵的应用。II单元特征值和特征矢量9小时特征值,特征矢量,Caley-Hamilton定理,矩阵的对角线化,矩阵的二次形式。第三单元差分计算-I 9小时连续的分化,Taylor's&McLaurin的系列,不确定形式,部分导数,Euler的定理,总导数IV差异单位计算9小时Jacobians&IT&Maxima and Maxima and Maxima and Maxima and Mixima of两次多变功能,该功能可实现lagrange的功能。单元V复杂分析9小时的复杂数字,Demovier定理,复杂函数,复杂函数的差异,分析功能,C-R方程,谐波功能教科书:1。B. S. Grewal的高级工程学数学(德里Khanna出版物)。